It is well-known that the magnification of an imaging lens changes with the object distance (distance from the object to the lens). However, it is less known that the magnification changes with the field angle as well (i.e, where in the field the object is placed). Additionally, the off-axis magnification can be asymmetric. In other words, the tangential and sagittal magnifications can be different.
This causes shape deformation for off-axis objects. For example, a golf ball off-axis may have an oval-shaped image. In this article, we discuss the concepts of “relative magnification” and “aspect ratio” useful for characterizing the shape deformation for off-axis objects. An understanding of this topic will help to optimize lens designs for machine learning applications.
For an on-axis object coming from infinity, the image size is the product of the lens effective focal length (EFL) and the angle (in radians) subtended by the object from the entrance pupil position. When this object is moved off-axis the image size depends on the lens mapping function h(θ), where h is the image height on the image plane and θ is the field angle. The derivative dh/dθ provides the tangential magnification (or meridional magnification). The definitions of tangential and sagittal orientation are shown in this figure.
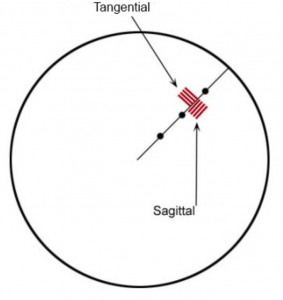
We can define the relative tangential magnification as the tangential magnification divided by on-axis magnification. In the special case where the lens mapping function is simply EFL*θ the tangential magnification is a constant, independent of the field angle (but the sagittal magnification is not). Such a lens is known as a f-θ lens. Other well-known forms of lens mapping function include: rectilinear, stereographic, equisolid angle, and orthographic (see this Wikipedia article).
It is worth noting that most real-world lenses do not follow these equations precisely. At Sunex we have developed a concept called “rectilinearity” as a generalized parameter to characterize the entire set of lens mapping functions. Please contact us if you are interested in learning more about this. Most lenses on the market today have axial symmetry. Based on this symmetry it can be shown that the sagittal magnification is h(θ)/sin(θ). The relative sagittal magnification is defined as the sagittal magnification divided by the on-axis magnification for axially symmetric systems. The ratio of relative tangential magnification to relative sagittal magnification is then defined as the “aspect ratio”. If the aspect ratio is 1 at all field angles, the shape of the object is invariant across the field of view of the lens. Such lens may help to improve the accuracy of machine learning algorithms.
Let us study a few special cases:
- The most common type of lens mapping function is known as rectilinear. The mapping function is based on a pinhole model where the image height is proportional to the tangent of the field angle. Examples of rectilinear lenses include most photographic lenses including smartphone lenses. Both relative tangential and sagittal magnifications increase with field angle, but not at the same rate. The tangential increase is faster resulting in an aspect ratio that also increases with the field angle. The practical effect is a person near the horizontal edge is stretched widthwise.
- A stereographic lens: Both tangential and sagittal magnification increases with the field angle but at the same rate. The aspect ratio is invariant across the field of view of a stereographic lens.
- A f-θ lens has constant tangential magnification. However, the sagittal magnification increases with field angle. As a result, the aspect ratio decreases with increased field angle. Off-axis objects become “elongated” sagittally.
- An equisolid angle lens has decreasing tangential magnification but an increasing sagittal one resulting in the product of the two being constant. Though the objects off-axis are deformed they occupy the same number of pixels in the image plane approximately. If the machine learning algorithm requires a minimum number of pixels across the field of view to function, an equisolid angle lens may be useful.
We have developed an online wizard to calculate the relative magnifications and aspect ratio at HFOV, VFOV, and DFOV points for all lenses with known rectilinearity. Try this wizard out !


